NuT

From README.md

NuT simulates neutrino transport. Currently, it runs in 1D, spherical and 3D Cartesian geometries (the mesh geometry and topology is carefully encapsulated, so adding another mesh requires a few hundred lines of interface implementation). Particles events are tallied in the lab frame, while scattering events (cross sections) are calculated in the co-moving frame.

---

Build and Dependency Information

Nut builds a library using cmake. It depends on the Random123 library.

NuT includes a small application to demonstrate the library, called bh-3. “bh” is for black hole—the application models the neutrino transport given a snapshot of the material state of a star collapsing to a black hole.

---

Analysis

---

Build and Run Information

Compiler = icpc (ICC) 18.0.1 20171018
Run Parameters = -n 114688 -c 2048 -I ../../test/data/p.2

---

Scaling

---

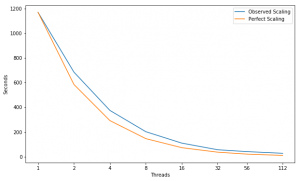
Hit Locations

---

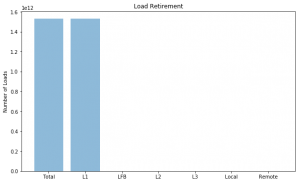
Intel Software Development Emulator

Intel SDE	NuT
Arithmetric Intensity	0.00924
FLOPS per Inst	0.0363
FLOPS per FP Inst	1.0
Bytes per Load Inst	6.76
Bytes per Store Inst	6.73

---

UOPS Executed

---

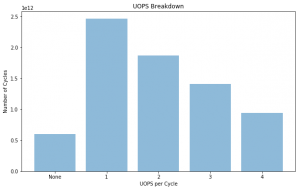
Experiment Aggregate Metrics

Threads (Time)	IPC per Core	Loads per Cycle	L1 Hits per Cycle	L1 Miss Ratio	L2 Miss Ratio	L3 Miss Ratio	L2 B/W Utilized	L3 B/W Utilized	DRAM B/W Utilized
1 (100.0%)	1.68	0.52	0.51	0.06%	0.43%	11.17%	0.31%	0.01%	0.01%
56 (100.0%)	1.66	0.50	0.50	0.06%	2.00%	29.86%	0.16%	0.02%	0.11%
112 (100.0%)	1.67	0.49	0.49	0.06%	2.31%	31.13%	0.14%	0.02%	0.10%

---

decide_event

Threads (Time)	IPC per Core	Loads per Cycle	L1 Hits per Cycle	L1 Miss Ratio	L2 Miss Ratio	L3 Miss Ratio	L2 B/W Utilized	L3 B/W Utilized	DRAM B/W Utilized
1 (57.3%)	1.65	0.52	0.52	0.09%	0.26%	12.33%	0.39%	0.01%	0.01%
56 (29.5%)	1.67	0.51	0.51	0.09%	1.35%	26.93%	0.38%	0.04%	0.15%
112 (22.3%)	1.68	0.51	0.51	0.09%	1.55%	28.60%	0.39%	0.05%	0.17%

 29     template <typename particle_t, typename mesh_t, typename opacity_t,
 30               typename velocity_t>
 31     event_n_dist
 32     decide_event( particle_t & p, // non-const b/c of RNG
 33                   mesh_t const & mesh,
 34                   opacity_t const & opacity,
 35                   velocity_t const & velocity)
 36     {
 37         static const size_t dim(particle_t::dim);
 38 
 39         typedef typename particle_t::fp_t fp_t;
 40         // Currently there are always  three top-level events considered.
 41         // Changing vector to std::array
 42         typedef std::array<event_n_dist,3> vend_t;
 43         typedef typename mesh_t::d_to_b_t d_to_b_t;
 44 
 45         vend_t e_n_ds;
 46 
 47         cell_t const cell  = p.cell;
 48         fp_t const tleft   = p.t;
 49         vec_t<dim> const x = p.x;
 50         Species const species = p.species;
 51 
 52         // compute distance to events, push onto vector
 53         // compute cross-section in comoving frame
 54 
 55         vec_t<dim> v = velocity.v(cell);
 56         // TO DO vector velocity
 57         vec_t<dim> vtmp(v);
 58         geom_t const eli  = p.e;
 59         vec_t<dim> const oli  = p.omega;
 60         // LT to comoving frame (compute interaction comoving).
 61         EandOmega<dim> eno_cmi = mesh_t::LT_to_comoving(vtmp,eli,oli);
 62         geom_t const eci  = eno_cmi.first;
 63 
 64         fp_t const sig_coll   = opacity.sigma_collide(cell,eci,species);
 65 
 66         fp_t const random_dev = p.rng.random();
 67         /* fp_t const ignored    =*/ p.rng.random(); // to keep pace with McPhD
 68 
 69         geom_t const d_coll     = (sig_coll != fp_t(0)) ?
 70             -std::log(random_dev)/sig_coll : huge;
 71 
 72         e_n_ds[0] = event_n_dist(Event::collision,d_coll);
 73 
 74         d_to_b_t dnf = mesh.distance_to_bdy(x,oli,cell);
 75         geom_t const d_bdy = dnf.d;
 76         e_n_ds[1] = event_n_dist(Event::boundary,d_bdy);
 77 
 78         geom_t const d_step_end = c * tleft;
 79         e_n_ds[2] = event_n_dist(Event::step_end,d_step_end);
 80 
 81         // pick (event,dist) pair with shortest distance
 82         event_n_dist closest = *(std::min_element(e_n_ds.begin(),e_n_ds.end(),
 83                                                   pair_min_2nd<event_n_dist>));
 84 
 85         // if needed, further resolve events
 86         if(closest.first == Event::collision)
 87         {
 88             // need to compute the comoving energy at the scattering site,
 89             // in order to compute the different cross sections there.
 90 
 91             typename mesh_t::coord_t const scat_site(
 92                 mesh.new_coordinate(x,oli,d_coll));
 93             vec_t<dim> const o_sct = scat_site.omega;
 94             EandOmega<dim> const eno_scat = mesh_t::LT_to_comoving(vtmp,eli,o_sct);
 95             fp_t const ecscat = eno_scat.first;
 96             closest.first =
 97                 decide_scatter_event(p.rng,ecscat,cell,opacity,species);
 98         }
 99         else
100         {
101             if(closest.first == Event::boundary)
102             {
103                 closest.first = decide_boundary_event(mesh,cell,dnf.face);
104             }
105             // compensate RNG if decide_scatter_event not called
106             p.rng.random();
107         }
108         return closest;
109     } // decide_event

---

apply_event

Threads (Time)	IPC per Core	Loads per Cycle	L1 Hits per Cycle	L1 Miss Ratio	L2 Miss Ratio	L3 Miss Ratio	L2 B/W Utilized	L3 B/W Utilized	DRAM B/W Utilized
1 (42.1%)	1.70	0.51	0.51	0.02%	0.46%	12.31%	0.20%	0.01%	0.00%
56 (21.7%)	1.71	0.50	0.50	0.02%	2.60%	29.96%	0.20%	0.03%	0.15%
112 (16.4%)	1.73	0.50	0.50	0.02%	2.40%	34.00%	0.22%	0.04%	0.16%

 46     template <typename ParticleT, typename MeshT, typename OpacityT,
 47               typename VelocityT, typename fp_t>
 48     void
 49     apply_event(ParticleT & p,
 50                 Event const & event,
 51                 geom_t const distance,
 52                 MeshT const & mesh,
 53                 OpacityT const & opacity,
 54                 VelocityT const & velocity,
 55                 Tally<fp_t> & tally,
 56                 fp_t const alpha = fp_t(2.0)
 57         )
 58     {
 59         cell_t const index = make_idx(p.cell,opacity.m_n_cells);
 60 
 61         stream_particle(p,distance,mesh);
 62 
 63         tally.accum_pl(distance);
 64 
 65         switch(event)
 66         {
 67         case Event::nucleon_abs:
 68             apply_nucleon_abs(p,tally);
 69             break;
 70         case Event::nucleon_elastic_scatter:
 71             apply_nucleon_elastic_scatter<ParticleT,Tally<fp_t>,VelocityT,MeshT>( p,
                                                                            tally,velocity);
 72             break;
 73         case Event::electron_scatter:
 74             {
 75                 fp_t const ebar = opacity.m_T.T_e_minus[index];
 76                 fp_t const e_e  = ebar;
 77                 // fp_t const e_e  = gen_power_law_energy_alpha2(ebar,p.rng);
 78                 apply_lepton_scatter<ParticleT,Tally<fp_t>,VelocityT,MeshT>(p,
                                                                        tally,e_e,velocity);
 79             }
 80             break;
 81         case Event::positron_scatter:
 82             {
 83                 fp_t const ebar = opacity.m_T.T_e_plus[index];
 84                 fp_t const e_e  = ebar;
 85                 // fp_t const e_e  = gen_power_law_energy_alpha2(ebar,p.rng);
 86                 apply_lepton_scatter<ParticleT,Tally<fp_t>,VelocityT,MeshT>(p,
                                                                        tally,e_e,velocity);
 87             }
 88             break;
 89         // case Event::nu_e_annhilation:
 90         //     break;
 91         // case Event::nu_x_annhilation:
 92         //     break;
 93         case Event::cell_low_x_boundary:
 94             apply_low_x_boundary(p,tally);
 95             break;
 96         case Event::cell_high_x_boundary:
 97             apply_hi_x_boundary(p,tally);
 98             break;
 99         case Event::escape:
100             apply_escape(p,tally);
101             break;
102         case Event::reflect:
103             apply_reflect(p,tally);
104             break;
105         case Event::step_end:
106             apply_step_end(p,tally);
107             break;
108         // error if we get to an unresolved collision or boundary
109         case Event::undetermined:
110             p.kill(Fates::UNDETERMINED_EVENT);
111             break;
112         case Event::boundary:   // fall through to next
113         case Event::collision:  //
114             // underresolved_event(event);
115             // break;
116         default:
117             p.kill(Fates::UNHANDLED_EVENT);
118         } // switch
119         if(event != Event::nucleon_elastic_scatter
120            && event != Event::electron_scatter
121            && event != Event::positron_scatter)
122         {
123             p.rng.random(); // compensate to maintain constant RNs/MC step
124         }
125         return;
126     } // apply_event

---

random

Threads (Time)	IPC per Core	Loads per Cycle	L1 Hits per Cycle	L1 Miss Ratio	L2 Miss Ratio	L3 Miss Ratio	L2 B/W Utilized	L3 B/W Utilized	DRAM B/W Utilized
1 (26.7%)	2.68	0.71	0.71	0.02%	0.57%	16.06%	0.17%	0.01%	0.00%
56 (22.8%)	2.67	0.70	0.70	0.02%	2.42%	30.93%	0.11%	0.02%	0.09%
112 (13.9%)	2.65	0.69	0.69	0.02%	2.71%	32.31%	0.15%	0.03%	0.12%

 39         double random() {
 40             double ret = m_bank[m_idx];
 41             if(m_idx == 0) m_idx++;
 42             else
 43             {
 44                 m_idx = 0;
 45                 this->advance();
 46             }
 47             return ret;
 48         }

---

stream_particle

Threads (Time)	IPC per Core	Loads per Cycle	L1 Hits per Cycle	L1 Miss Ratio	L2 Miss Ratio	L3 Miss Ratio	L2 B/W Utilized	L3 B/W Utilized	DRAM B/W Utilized
1 (26.4%)	1.22	0.40	0.40	0.02%	0.52%	9.59%	0.15%	0.01%	0.00%
56 (13.6%)	1.23	0.40	0.40	0.02%	2.75%	27.17%	0.16%	0.03%	0.14%
112 (10.3%)	1.25	0.40	0.40	0.02%	2.61%	30.30%	0.17%	0.03%	0.14%

 35     template <typename Mesh_T, typename Particle_T, typename fp_t>
 36     void stream_particle(Particle_T & p, fp_t const d, Mesh_T const & mesh)
 37     {
 38         typename Mesh_T::coord_t newcoord =
 39             mesh.new_coordinate(p.x,p.omega,d);
 40         p.x     = newcoord.x;
 41         p.omega = newcoord.omega;
 42         p.t     = p.t - d / c;
 43         return;
 44     } // stream_particle

---

Opacity::sigma_collide

Threads (Time)	IPC per Core	Loads per Cycle	L1 Hits per Cycle	L1 Miss Ratio	L2 Miss Ratio	L3 Miss Ratio	L2 B/W Utilized	L3 B/W Utilized	DRAM B/W Utilized
1 (10.1%)	1.81	0.59	0.59	0.26%	0.12%	6.44%	0.88%	0.01%	0.01%
56 (5.3%)	1.81	0.58	0.58	0.26%	0.91%	25.69%	0.81%	0.06%	0.19%
112 (4.0%)	1.85	0.58	0.58	0.26%	1.19%	31.74%	0.83%	0.08%	0.22%

 46         /* total collision opacity */
 47         fp_t sigma_collide( cell_t const & cell,
 48                             fp_t const e_nu,
 49                             Species const nu_species) const {
 50             cellOK(cell,m_n_cells);
 51             nrgOK(e_nu);
 52             fp_t s_coll =
 53                 sigma_N_total(cell, e_nu) +
 54                 sigma_lepton(cell,e_nu, nu_species);
 55             return s_coll;
 56         } // sigma_collide

---

Sphere_1D::distance_to_bdy

Threads (Time)	IPC per Core	Loads per Cycle	L1 Hits per Cycle	L1 Miss Ratio	L2 Miss Ratio	L3 Miss Ratio	L2 B/W Utilized	L3 B/W Utilized	DRAM B/W Utilized
1 (9.5%)	1.29	0.46	0.46	0.12%	0.10%	10.20%	0.83%	0.01%	0.00%
56 (4.8%)	1.34	0.47	0.46	0.12%	0.78%	26.17%	0.81%	0.05%	0.15%
112 (3.7%)	1.34	0.46	0.45	0.12%	0.81%	35.02%	0.80%	0.05%	0.15%

175         d_to_b_t
176         distance_to_bdy(vec_t<dim> const x, vec_t<dim> const omega,
177                         cell_t const cell) const noexcept
178         {
179             // cellOK(cell);
180             extents_t extents = this->cell_extents(cell);
181             return dist_to_bdy_impl(x.v[0],omega.v[0],extents.first,extents.second);
182         } // distance_to_bdy
{...}
185         static
186         d_to_b_t
187         dist_to_bdy_impl(geom_t const x, geom_t const omega,
188                          geom_t const rlo, geom_t const rhi) noexcept
189         {
190             geom_t const rhisq = rhi * rhi;
191             geom_t const rlosq = rlo * rlo;
192             geom_t const xsq   = x * x;
193 
194             // need to (1-2) check for intersection with the two spheres that
195             // bound the current cell, and (3) choose the first intersection
196             // along the direction of travel.
197 
198             // 1. Compute intersections with outer sphere
199 
200             // get Tan(theta) from inverting omega=Cos(theta). We work with a
201             // reduced polar angle, "abs_theta", in the range 0 <= theta <= pi/2,
202             // then select the correct intercept between the line that the
203             // particle travels and the spherical shell using the full value of
204             // theta.
205             geom_t const theta = std::acos(omega);
206             geom_t const abs_theta = (theta > pi/2) ?  pi - theta : theta;
207             geom_t const t = std::tan(abs_theta);
208             geom_t const tsq = t*t;
209             geom_t const tcub = tsq * t;
210             geom_t const one_plus_tsq = 1 + tsq;
211             geom_t const one_on_one_plus_tsq = 1.0/one_plus_tsq;
212             // the determinant is r^2 + r^2 * t^2 - x^2 * t^2. Include the
213             // square root and divide by 1+Tan(theta)^2 for convenience here.
214             geom_t const dethi =
215                 std::sqrt( rhisq * one_plus_tsq - xsq * tsq) * one_on_one_plus_tsq;
216             // These parts don't depend on the radius of the sphere.
217             geom_t const xterm1 = x*tsq*one_on_one_plus_tsq;
218             geom_t const yterm1 = -x * t + x * tcub * one_on_one_plus_tsq;
219             // These are the two intersection with the outer sphere
220             geom_t const xhip = xterm1 + dethi;
221             geom_t const yhip = yterm1 + t * dethi;
222             geom_t const xhim = xterm1 - dethi;
223             geom_t const yhim = yterm1 - t * dethi;
224             // if the polar angle is less than pi/2, we want the solution
225             // that adds the determinant.
226             geom_t const xhi = (theta < pi/2) ? xhip : xhim;
227             geom_t const yhi = (theta < pi/2) ? yhip : yhim;
228             geom_t const d_hi = std::sqrt( (xhi-x)*(xhi-x) + (yhi*yhi));
229 
230             // 2. Look for intersection with inner sphere
231             // There is if the absolute value of the polar angle is
232             // less than atan(1/(b^2 -1)), where b = x/r_lo. In this case, we can
233             // just use the "plus" solution, since that's always the closest.
234             // Note that you may want to know a real solution exists before
235             // computing it--complicates branch removal.
236             geom_t d_lo = huge;
237             if(rlo > 0.0)
238             {
239                 geom_t const b = x/rlo;
240                 // if the particle is on the inner sphere, and headed inward...
241                 if(soft_equiv(b,1.0,1e-11))
242                 {
243                     if(theta > pi/2)
244                     {
245                         d_lo = 0.0;
246                     }
247                 }
248                 else
249                 {
250                     geom_t const tan_theta_lim = std::sqrt(1/(b*b - 1));
251                     geom_t const theta_lim = std::atan(tan_theta_lim);
252                     if(abs_theta <= theta_lim and theta > pi/2)
253                     {
254                         geom_t const detlo =
255                             std::sqrt( rlosq * one_plus_tsq - xsq * tsq)
256                             * one_on_one_plus_tsq;
257                         geom_t const xlo = xterm1 + detlo;
258                         geom_t const ylo = yterm1 + t * detlo;
259                         d_lo = std::sqrt( (xlo-x)*(xlo-x) + ylo*ylo);
260                     }
261                 }
262             }
263             // now select the shorter distance and the corresponding face
264             geom_t d_bdy = huge;
265             cell_t face  = -1;
266             if( d_hi < d_lo)
267             {
268                 d_bdy = d_hi;
269                 face  = 1;     // will intersect outer sphere
270             }
271             else
272             {
273                 d_bdy = d_lo;
274                 face  = 0;     // will intersect inner sphere
275             }
276             d_to_b_t d2b;
277             d2b.d = d_bdy;
278             d2b.face = face;
279             return d2b;
280         } // dist_to_bdy_impl

---

Sphere_1D::LT_to_comoving

Threads (Time)	IPC per Core	Loads per Cycle	L1 Hits per Cycle	L1 Miss Ratio	L2 Miss Ratio	L3 Miss Ratio	L2 B/W Utilized	L3 B/W Utilized	DRAM B/W Utilized
1 (8.4%)	1.11	0.38	0.38	0.01%	0.77%	80.92%	0.07%	0.01%	0.01%
56 (4.3%)	1.12	0.37	0.38	0.01%	4.09%	25.08%	0.09%	0.03%	0.13%
112 (3.3%)	1.15	0.37	0.36	0.01%	3.98%	23.53%	0.11%	0.03%	0.15%

283         static
284         inline
285         EandOmega<1>
286         LT_to_comoving(vec_t<1> const v_lab,
287             geom_t const & e_lab,
288             vec_t<1> const & omega_lab) noexcept
289         {
290             return spec_1D::LT_to_comoving_sphere1D(v_lab.v[0],e_lab,omega_lab);
291         } // LT_to_comoving

 35         EandOmega<1>
 36         inline
 37         LT_to_comoving_sphere1D(geom_t const v_lab,
 38                                 geom_t const e_lab,
 39                                 vec_t<1> const omega_lab) noexcept
 40         {
 41             geom_t const voc       = v_lab/c;
 42             geom_t const onemovoc  = (1.0 - omega_lab.v[0] * voc);
 43             geom_t const e_com     = e_lab * gamma(v_lab) * onemovoc;
 44             vec_t<1> omega_com;
 45             omega_com.v[0] = (omega_lab.v[0] - voc) / onemovoc;
 46             return EandOmega<1>(e_com,omega_com);
 47         } // LT_to_comoving_sphere1D